
These three theorems, known as Angle - Angle (AA), Side - Angle - Side (SAS), and Side - Side - Side (SSS), are foolproof methods for determining similarity in triangles. To prove that the triangles are similar by the SAS similarity theorem, it needs to be proven that I measures 60°. This theorem is also known as the AAA similarity theorem. SAS Similarity (Side-Angle-Side) Criterion SAS Similarity Criterion states that If two sides of one triangle are in proportion with the two sides of the other triangle and also one included angle between the sides is equal to the included angle of another triangle then the two triangles are similar. Similar triangles are easy to identify because you can apply three theorems specific to triangles. In other words, if two angles are equal in measure, then they are equal in shape. Note: Note that in similar triangles, each pair of corresponding sides are proportional.Īlso, if two triangles are congruent, therefore they are similar (although the converse is not always true). $\Rightarrow$\, since we know that if two triangles are congruent, therefore they are similar.
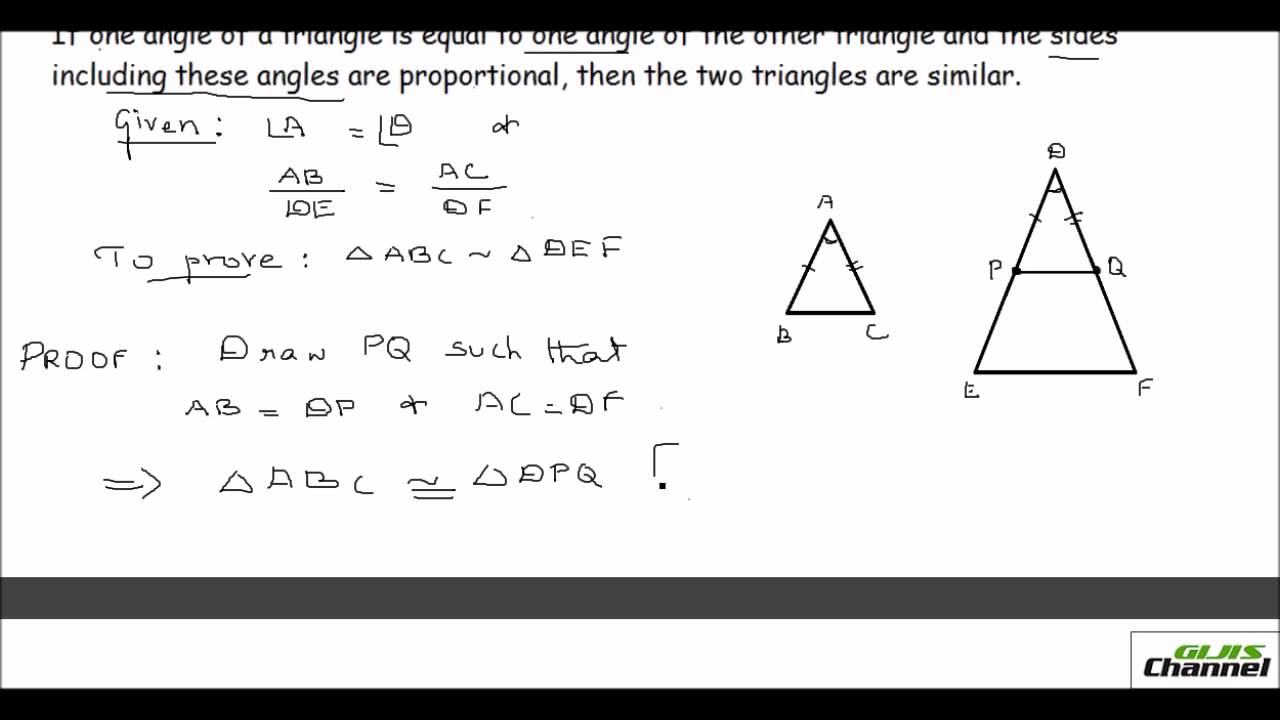
Therefore the triangles are similar with the SAS Triangle Similarity.
---class-10---if-one-angle-of-triangle-is-equal-to-one-angle-of-the-other.jpg)
#SAS SIMILARITY THEOREM SOFTWARE#
Therefore, by the SAS Congruency Criterion, Lesson Summary: Students will construct two similar triangles using Geometry software and discover the Side-Angle-Side Similarity. The angle in between is congruent as indicated by the same-colored arc on the angle.


 0 kommentar(er)
0 kommentar(er)
